Astronomy 101 Problem Set #7 Solutions -- Fall 2005

Astronomy 101
Problem Set #7 Solutions

Problem #1: Bianca is a moon of Uranus. Bianca has a circular orbit whose radius is 5.92 x 10⁴ km, and its orbital period is 0.435 days.

a) Calculate Bianca's speed in its orbit around Uranus.

b) From this information, calculate the mass of Uranus.

Solution: Speed is defined as distance per time, e.g., "miles per hour," "meters per second," etc. In this problem (as in the Exam!) we have both a distance and a time, so calculating the speed does not require some fancy formula.

Let's start with a simple diagram of the situation:

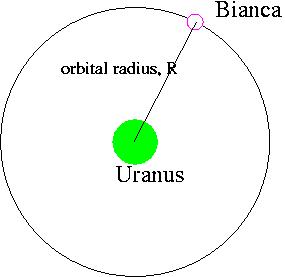
Here, the moon Bianca orbits in a circle around Uranus. How far does it travel to complete one lap around Uranus? Not the radius of its circular path, but the circumference, which is

circumference = 2 pi R
= 2 x 3.14 x (5.92 x 10⁴ km)
= 3.72 x 10⁵ km

Now this is a perfectly good distance in perfectly good units, but since I know what's coming later, I'm going to convert it now into the more standard unit of meters:

3.72 x 10⁵ km x (1000 m/1 km)
= 3.72 x 10⁸ m

Now, how long does it take for Bianca to complete one lap around Uranus? That's the period, which is:

period = 0.435 days
= 0.435 days x (24 hours/ 1 day) x (60 minutes/ 1 hour) x (60 seconds/ 1 minute)
= 3.76 x 10⁴ seconds

OK, so now we have the distance traveled in one lap and the time it takes to travel this distance. So,

speed = distance/time
= 3.72 x 10⁸ m / 3.76 x 10⁴ seconds
= 9.9 x 10³ m/s, or 9900 m/s

Now for part b), where we need to find the mass of Uranus. We have the speed from part a) above, and we know that Bianca travels in a circular orbit. From these two pieces of information, we can calculate the acceleration Bianca must feel, since, for any object traveling in a circle,

acceleration = speed²/radius
= (9900 m/s)²/(5.92 x 10⁷ m)
= 1.65 m/s²

(Note that I converted the radius to meters by 5.92 x 10⁴ km x (1000 m/ 1 km) = 5.92 x 10⁷ m.)

This is the acceleration that Bianca must experience in order to travel in a circle, and that acceleration must be caused by the gravity of Uranus (there really isn't any other choice). Newton told us that the acceleration due to gravity is:

acceleration = G x Mass (of planet)/ distance²

Here, notice that the mass involved is not the mass of Bianca, but the mass of the object responsible for the gravity (i.e., Uranus). We can rearrange this expression as follows and insert the acceleration we calculated for Bianca in its orbit, :

Mass = acceleration x distance²/ G
= 1.65 m/s² x (5.92 x 10⁷ m)² / 6.67 x 10^-11m³/kg s²
= 8.67 x 10²⁵ kg

Now the units do in fact work out, but only if you used appropriate units for the acceleration and distance so that they cancel properly with the units for G. That's why it's important in general to calculate parameters in "standard" units of meters, kilograms, and seconds.

Problem #2: Mercury's iron core occupies 40% of the volume of the planet, but accounts for 60% of its total mass. Using the mass and radius of Mercury in the back of your textbook, calculate the average density of the other part of Mercury, that is, the rocky outer layers.

Solution: Density is mass per volume, so here we just need to figure out the mass and volume of the outer part of Mercury. Since we're given the mass and volume of Mercury's inner iron core, we really only need to subtract these values from the total mass and volume of Mercury to get the value we need.

Looking in the back of our textbook, I find that radius of Mercury is 2439 km, or,

2439 km x ( 1000 m/1 km) = 2.439 x 10⁶ m

From this value, I can calculate Mercury's total volume (assuming it's a sphere),

volume = 4/3 x pi x radius³
= 1.33 x 3.14 x (2.439 x 10⁶ m)³
= 6.07 x 10¹⁹ m³

Now the volume of Mercury's iron core is 40% of its total volume, so the volume of what's left must be 60% of Mercury's total volume, so

volume of outer part = 0.6 x 6.07 x 10¹⁹ m³
= 3.64 x 10¹⁹ m³

Now, the mass of Mercury is 3.3 x 10²³ kg (from the back of our text), and the mass of its iron core is 60% of the total mass, so the mass of what's left must be 40% of Mercury's total mass,

mass of outer part = 0.4 x 3.3 x 10²³ kg
= 1.32 x 10²³ kg

Now, density is just mass per volume, or

density = mass/volume
= 1.32 x 10²³ kg /3.64 x 10¹⁹ m³
= 3.63 x 10³ kg/km³, or 3630 kg/km³

This is approximately the density of rocks in the Earth's crust, which makes sense since we think the outer part of Mercury has a similar rocky crust.

Problem #3: The maximum elongation of Venus (that's the largest angular separation between Venus and the Sun, viewed from Earth) is 46 degrees.

a) Draw a diagram indicating the position of the Sun and Earth, the orbit of Venus, and its location when it appears at its maximum elongation.

b) From this diagram (and the knowledge that the Earth-Sun distance is 1 A.U.), calculate the radius of Venus' circular orbit.

Solution: The largest angle between the direction to the Sun and the direction to Venus will occur for the following geometry,

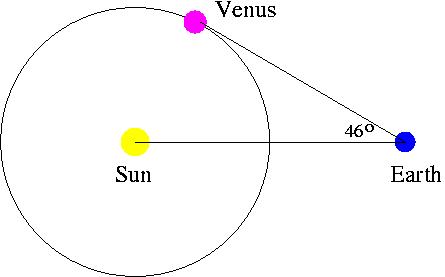
where the line from the Earth to Venus just touches the circle that defines Venus's orbit (mathematicians would say that this line is "tangent" to the circle). We can then make a triangle with vertices at the Earth, Venus, and the Sun,

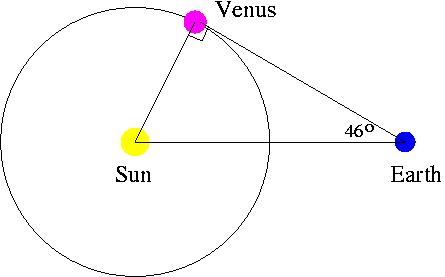
where the angle at Venus is a right angle (i.e., 90 degrees). Why this one and not the one at the Sun? Look at the picture. If the angle at the Sun were a right angle, Venus would be at the "top" of its orbit in the picture. But clearly, the elongation is larger when Venus's just a little bit closer to the Earth than when it's at the top. So the angle at the Sun must be slightly smaller than 90 degrees.

The angle at Venus is 90 degrees because the tangent line is always perpendicular to the radius, as drawn at left below:

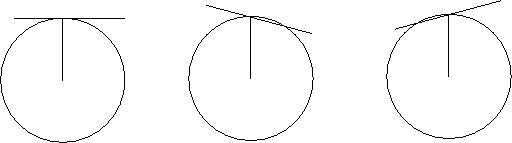
If the line weren't perpendicular to the radius line, it would "dig in" to the circle and wouldn't be tangent (see other two figures above).

So, from the geometry of the Sun-Venus-Earth triangle, and knowing SOHCATOA, we get that

Sin(elongation) = Sun-Venus distance/Sun-Earth distance = Sun-Venus distance/ 1 A.U.

so,

Sun-Merc distance = 1 A.U. x Sin(46 degrees) = 0.72 A.U.