EE 329
Prof. Rich Kozick
Spring, 1997

Signals in the Time Domain and the Frequency Domain

The important idea from class on Friday, January 24 is to understand how to interpret the complex numbers that arise in the frequency domain (Fourier transform) representation of signals. We looked at four signals, and their representations with real sines/cosines and complex exponentials:

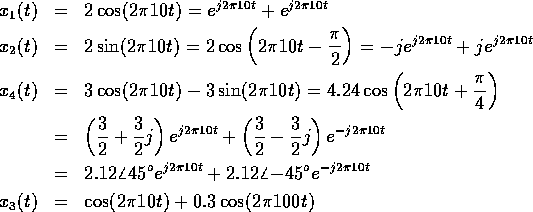
The complex coefficient D on the ``positive frequency'' exponential contains the following information: (refer to for illustrations)

• The real part is the amplitude of the cosine component.
• The imaginary part is the amplitude of the sine component.
• The magnitude |D| is the amplitude of the sinusoid.
• The angle is the phase shift of the sinusoid, relative to a cosine.

Note also that the complex coefficient on the ``negative frequency'' exponential is the complex conjugate of the positive frequency coefficient. This is always true for real-valued time signals. You can think of it like this: two complex exponentials are required to create a real sinusoid according to Euler's formula .

Thus the complex value of a frequency component tells you the amplitude and the phase shift of the corresponding sinusoid that composes the time signal.

 
• About this document ...