| <Previous | January | February | March | April | May | Next> |
Lecture 6: Ampere's Law
February 6, 2025
Reading Assignment
- Read: 26.8
- Study: Eq 26.17; Exs 26.6, 26.7, 26.8; Fig 26.34
Objectives
- (Continuing objective) Describe applications of the concepts of electricity and magnetism to everyday “real-life” situations.
- Distinguish and correctly use the expressions for the magnetic field for each of these special situations: (a) at the center of a circular loop or finite arcs of a circular loop; (b) inside and just outside the central region of a very long solenoid, (c) outside a wire segment or long straight wire. Use these and superposition to find the total B-field due to a combination of sources.
- Use Ampere's Law to relate the loop integral of \(\vec{B}\) (circulation) to the net encircled current.
- Use Ampere's Law to calculate the magnetic fields due to symmetric steady currents.
Homework
- Friday's Assigned Problems:
A21, A22, A111, X3 (below), X4 (below); CH 26: 8, 33, 67
Problem X3 A solenoid used in a plasma physics experiment is 10 cm in diameter, is 1.0 m long, and carries a 35 A current to produce a 100 mT magnetic field. (a) How many turns are in the solenoid? (b) If the solenoid resistance is $2.7\,\Omega$, how much power does it dissipate?
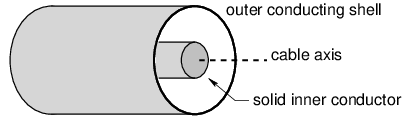Problem X4 A coaxial cable (see figure) consists of a 1.0 mm
diameter inner conductor and a hollow outer conductor with diameter
1.0 cm. A 100 mA current flows down the inner conductor and back
along the outer conductor. Find the magnetic field strength (a)
0.10 mm, (b) 4.0 mm, and (c) 2.0 cm from the cable axis.
Answers: X3 (a) 2300, (b) 3.3 kW; X4 (a) $8.0\times 10^{-6}\,\text{T}$, (b) $5.0\times 10^{-6}\,\text{T}$, (c) $0$
- Monday's Hand-In Problems from Lecture 6:
X7; CH 26: 34, 68, 70
Note: this is only the second half of the hand-in set.
Problem X7 The coaxial cable shown in Problem X4 consists of a solid inner conductor of radius $a$ and a hollow outer conductor of radius $b$. The two carry equal but opposite currents $I$, uniformly distributed. Find expressions for the magnetic field as a function of radial position $r$ (a) within the inner conductor, (b) between the inner and outer conductors, and (c) beyond the outer conductor.
Lecture Materials
- Click here for the Lecture overheads. Answers: CT1 - 1; CT2 - 3; CT3 - 3; CT4 - 4; CT5 - 6
Videos of example problems
To see the problem statement, click on the link below. To play the video example, click on the underlined words "Video Demonstration" near the top of the page with the problem statement.Pre-Class Entertainment
- It's Too Late - Carole King
- Me and Julio Down by the Schoolyard - Paul Simon
- Better Together - Jack Johnson
- Eet - Regina Spektor
- Subterranean Homesick Blue - Bob Dylan
Assigned Problems Guide
- A21: toy kit problem. Make an electromagnet. This one is fun!
- A22: medium-quick. Use the solenoid expression for the magnetic field, but think about what $n$ is.
- A111: long Ampere's law problem. The circulation will be more or less the same for each part (different r's), but think carefully about the encircled current.
- X3: medium-long. Here you know the B field, and you need to back out of that how many turns are in the solenoid. And don't forget the relation between power, voltage, and current.
- X4: another long Ampere's law problem. A lot like A111. The work goes into figuring out the encircled current for each case.
- 26-8: medium-quick (once you understand it). Think about how to relate field lines and circulation.
- 26-33: quick. Use Ampere's law to find the unknown current.
- 26-67: medium. Another Ampere's law problem, and again the main work is finding the encircled current. But this one is less involved than A111 and X4.